atalanta fugiens xx1 (21)
Atalanta Fugiens Emblem XX1 (21)
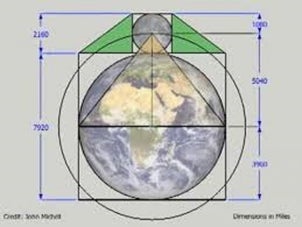


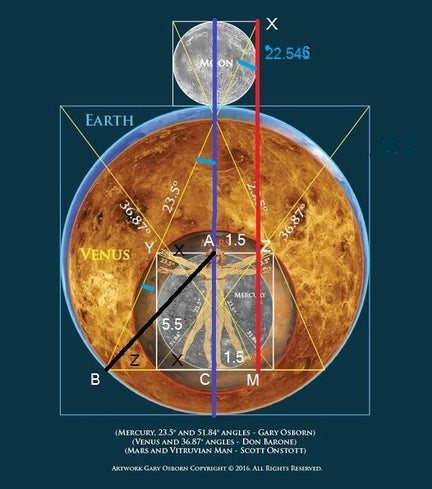
The first diagram is very much John Michell in his prime: Moon atop Earth. The Earth's diameter is about 7926 miles and the Moon's about 2160. John wrote of "canonical" numbers; in this case the Earth became 7920 and the Moon exactly 2160.
These numbers are divisible by 720, so the relationship can be expressed to scale as a 3 x 3 Moon square sitting on top of an 11 x 11 Earth square. You get the idea in the second and fourth diagrams.
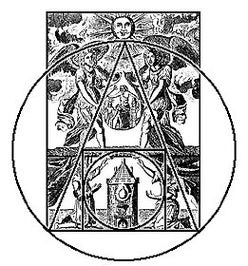
Enter Gary Osborn. He wondered about Maier's 1617 Atalanta Fugiens Emblem XXI (diag. 3, above), an expression of the Philosopher's Stone mantra - "Make of a man and woman a circle; then a quadrangle; out of this a triangle; make again a circle, and you will have the Stone of the Wise. Thus is made the stone, which thou canst not discover, unless you, through diligence, learn to understand this geometrical teaching." JB Craven, "Count Michael Maier", p.90 https://en.wikipedia.org/wiki/Philosopher%27s_stone
Could the larger circle indicated represent the Earth and the smaller one inside be Mercury, mused Gary? And why didn't the pyramid reach the top of the outer circle, most especially since the accompanying Maier epigram reads it "should touch the circle on every side"? Gary thought the angle at the pyramid top was 23.5 X 2 degrees. This value has some possible import as it is the angle of the Earth's equator to the tropics (the sun's journey through half the year = 2 x 23.5 = 47 degrees, Cancer to Capricorn and vice versa) and 23.5 degrees is also the value commonly given for the Earth's axial tilt.
Note 1: authors such as Gary Osborn, Jeff. Nisbet, J. Douglas Kenyon et al cite Frank C Higgins, Ancient Freemasonry: An Introduction to Masonic Archeology, 1919, as the source for the 23.5 degree angle and its double, 47 degrees, being two of Freemasonry's "Cosmic Angles". And elsewhere, in the same tropic and tilt context, Higgins does make quite some mention of 23.5 and 47 degrees.
Note 2: each Emblem is accompanied by an Epigram. Epigram(ma) XXI - quoted above - translates literally as (and my thanks here to Peter Jones and the Hull "Classics for All" organisation):
Let a female and male become for you one circle, from which
Let arise a quadratic shape having equal side[s].
From here extend a triangle, which in every part
returns onto a round sphere: then a Stone will have emerged.
If so great a matter does not soon come to your mind,
If you master the truth of geometry, you will know everything.
Now Maier's triangle self-evidently does not do what the Epigram says it should - have all three corners touching the outer circle. Notice how similar it is, though, to the triangle on the right (diag. 4, top). I was part of the Maier discussions (on GHMB) - all those years ago - and so I sat up and took notice when a new idea popped up I hadn't seen before: Venus. The idea came from Gary's erstwhile friend, Don Barone, and his observation answered Gary's wonder about the pyramid not reaching the circle: the Earth and Venus are quite similar in size, with Venus just a tad smaller. The distance from the bottom of the Earth to the top of the pyramid was Venus?
Now I'd messed about with the maths in the intervening years and had derived - largely by trial and error - a working new value for the pyramid slope of about 22.5 degrees (to Gary's 23.5 - as indicated above, diag. 4). This was because Maier has (at least) two constraints: the top of "Mercury" has to be on the equator and "Mercury" has to be in a square (and not a rectangle) since it is a circle touching all 4 sides - but noting "quadrangle" can mean both square and rectangle (but also noting that the translation provided by Peter Jones expressly talks of equal sides). In fact, and given these two constraints, I'd trigged it all out to quite a few decimal places and knew that my numbers were pretty much spot on. And that meant I could provide a more-or-less exact value for the gap between the top of the pyramid and the circle above it. And that, in turn, meant I could quantify the diameter of Venus. The result? 99.44% accurate.
I gave it some more thought while out walking on a lovely afternoon the next day. Two things occurred: what about Mars and why not simplify? Firstly Mars: well, it turns out that 1/2 Venus (which I now knew) + Mars = Earth. Well almost - there's about 40-50 or so miles in it. Over nearly 8000 miles, though, about 40-50 miles or so is quite tiny (especially when one square side = 720 miles, the error being about 1/16th of that). Anyway, the gap between the centre of Venus and Earth's top could now also be quantified at 99% + accurate. We had Mars! Only "Mercury" was a comparative weakness at just 96.35% accurate.
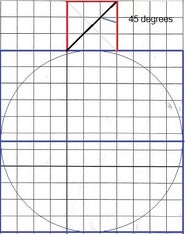
So, on to my second idea, simplification: I said the angle was "about" 22.5 degrees. What if I just used 22.5 exactly (@ 99.796 accuracy to the trigged model)? Forget all the trig. and start again with just ruler, pencil and compass. I've shown how to find a 45 degree angle in diagram 2 (top): you halve a 90 degree angle. Next you - and here comes a technical term - bisect that 45 degree angle:
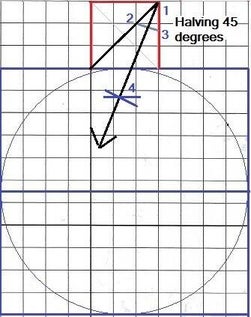
You find 4 from 2 and 3 using compasses, as you find 2 and 3 from 1 (Moon square top right). Draw a straight line from 1 through 4 and you have halved the 45 degree angle. Then you just develop through the "Equator" and on down to the circle (diag. 4, top). Repeat this process from Moon square top left.
Where the lines cut the Earth's Equator are the two top corners of the "Mercury" square. Joining up where those lines cut through the bottom of the Earth circle gives you a line providing the "Mercury" square's bottom. You just need to drop two verticals from the two top corners you just found.
For Venus, you use compasses again to find its centre - and that gives you Mars. You don't even need a grid, just an ruler, to measure 3 x 3 atop and centre of 11 x 11 ... et voila: 4 planets and the Moon delivered geometrically, fairly accurately, mostly, and fairly, constructionally, simply!
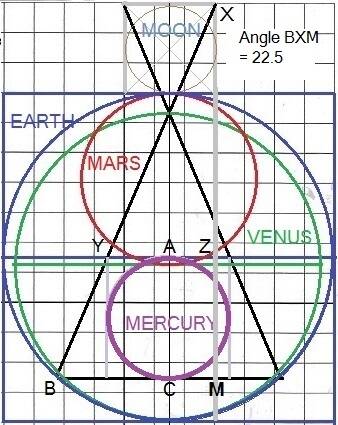
Am I the first person to ever look at this? The Venus and Mars values are incredibly accurate derived from that small gap - which is just like Maier's, 1617.
Note 1: The model scale is 720:1. Changing scales makes it possible to add Jupiter and Saturn. At 7200 : 1, Jupiter would be 12 and Saturn 10. At 72000 : 1, the Sun would be 12. All ("canonical" values) are divisible by 8 (and some by 9 - and thus 72). The lettering on the diagram is the basis for the trig.
Note 2: someone else identified 22.5. Cloister, GHMB, back in 2018:
https://grahamhancock.com/phorum/read.php?1,1163093
Note 3: https://grahamhancock.com/phorum/read.php?1,326732,326885
It's 2013 and Don Barone (Ahatmose) et GHMB al on the subject. Notice that the Mercury square does not conform to Maier at 23.5 degrees. Don's "thread" was started in response to Gary Osborn earlier floating the idea.
Note 4: back in 2013 (GHMB), I proposed an alternative to Mercury. I thought I noticed phi: "Mercury (3030 diam.)/ Earth (7920-9 range) = circa 0.38214-0.38257. But 1 - phi = 0.381966." A (today) correction is Mercury at 3031.9/7926 = 0. 382526. Still close! Just 56/100000ths or so in it.
Note 5: Don Barone was kind enough - and my thanks - to send me links to his study of Emblem XXI:
https://ahatmose2002.proboards.com/thread/796/solution-philosophers-stone
The outcome is strikingly similar to my own but has an apex angle of 2 X 22.62 degrees (to my 2 X 22.546). This angle derives from tan 2.5/6 in his final diagram. Whilst strikingly similar - @ 99.67% - the use of Don's angle would necessarily jettison Gary Osborn's 11 X 14 grid theory (based on Michell) and the "insights" gained from that. Mind - you'd never actually notice if you drew it that way, I'll hazard!
I have to stress the the whole initial insight was Gary Osborn's - and it was a real pleasure just to be "along for the ride"!
Footnotes: I use this area for on-going conversations with myself and others re. developing/exploring.
The model has constraints: only an angle of about 22.545-6 degrees will work with the Michell Earth-Moon values and layout + Maier's outer circle centre (but with a nod, perhaps, to the tilt idea - see below). Model values approx. / e&oe:
| Actual | /8 and/or /9 | 22.545 degrees | % model to actual | |
|---|---|---|---|---|
| Earth (Eq.) | 7926 | 7920 | (7920) | 99.92 |
| Moon (mean) | 2159.2 | 2160 | (2160) | 99.89 |
| Mercury | 3031.9 | 3032 | 2921.5 | 96.35 |
| Mars | 4212.3 | 4212 | 4180.9 | 99.25 |
| Venus | 7520.8 | 7520 | 7478.4 | 99.44 |
Gary Osborn's idea with some extra input from Don Barone and Scott Onstott. This idea cannot work - given the Maier constraints - but it's close (and you will see I added my angle and methodology - the purple, red and black lines and the similar triangle angles):
Here's someone (Claus Furstner) obeying Maier in all but one detail:

The original is on the left, Plate 2 of 15, Mutus Liber, Altus, 1677 (it's hard to see but the left and right characters in the "teardrop" have sun, left, and moon, right, on their heads). The overlay by Claus Furstner was @ https://tarotmutusliber.wordpress.com/2010/06/18/hello-world/ and here http://members.ozemail.com.au/~clauspat/stonea.htm#05 Note triangle touches the top, as Furstner quite rightly claims it should, based, that is, upon Epigram XXI.
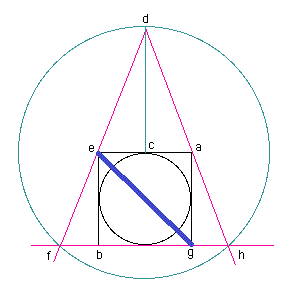
And this is Claus Furstner's idea as expressed on the "Altus", above, and on the Maier Epigram accompanying Atalanta Fugiens Emblem XXI. It looks very elegant, remembering he stresses that e-a is part of the outer circle's diameter and, thus, c is that circle's centre, with e-a-g-b as a (perfect) square. You build it, he explains, like this: 1. draw the square e-a-g-b; 2. draw the diagonal e-g; 3. e-g equals c-d (he says), so you now have the triangle d-e-a (and, I note, the angle d-e-c); extend d-e, d-a and b-g to find f and h. Et voila - the Philosopher's Stone.
But is it right? The maths is simple: let e-a = 2, giving us e-c = 1. e-g is sq. rt. of 8 (sq rt. of 2sq. + 2 sq. = 4 + 4 = 8). So d-c = Sq. rt. of 8, making the angles d-e-c and e-f-b Sq. Rt. 8/1 = Sq. Rt. 8 = (tan) 2.828427 = 70.5288 degrees ... roughly. So e-d-c is about 19.4712 degrees.
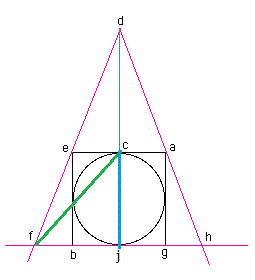
Now we know c-j = 2. So d-j = Sq. Rt. 8 + 2. And we know the angle d-f-h (because it is the same as d-e-c). So we can work out f-j: f-j = (Sq. Rt. 8 + 2)/tan 70.5288 = 1.7071. Given we have c-j = 2 and f-j = 1.7071, we can apply Pythagoras - the square on the hypotenuse = the sum of the squares of the other two sides. So f-c sq. equals 2 sq. + 1.7071 sq. = 6.91419 so f-c = 2.6295 .... But we already knew f-c. It's the same as d-c (both are radii of the same circle) = Sq. Rt. 8 = 2.828427. So, lovely idea, Claus, but it just doesn't work! It would work if e-c = 1 and c-d = 2.659. The angle e-d-c (f-e-j/f-d-j) would be 20.61 degrees. And it's not what Maier actually drew, of course, anyway!
Lastly ... Design weaknesses:
1. Firstly, "canonical numbers" - has little effect on outcomes.
2. Mercury - Maier's pyramid is a tad skewed. My offering isn't. By sacrificing a Maier constraint slightly the model can be tweaked to accommodate a more accurate value for Mercury without affecting the other derived values for Venus and Mars. 22.5 degrees left of vertical combined with, say, 24.5 degrees right of it - an angle of 47 degrees - would deliver a highly accurate "equatorial" value (at 99.75%):

Skewing the model: we now have a Mercury value of 3039.35 across but not down (v. 3031.9 = 99.755% accurate), valued by the top of the quadrangle (the shape Maier says it is). But we've not lost the critical Earth centre point C. And the Maier quadrangle is similarly skewed (as witnessed in Atalanta Fugiens XXI, above). We also have Gary's 47 pyramid apex (23.5 X 2). But we have lost the integrity of a square for Mercury (and Mercury is not oblate, it's round).
Geometrically, you could, perhaps, keep the 22.5 and then use the Maier protractor (the sea-shell looking thing in diag. 3, top) to add a 24.5 approximation.* I note that there is evidence of the existence of something similar to a protractor in 1589 AD and earlier, possibly, c/o one Thomas Blundeville:
https://lifethroughamathematicianseyes.wordpress.com/2017/01/14/history-of-protractors/
We are in 1617 AD.
* Or you could just guess, of course. A small increase in the cdy angle (B) will create a skew and - to a point - bring you closer to a more accurate "Mercury" value (true to the range 22.5-26.5). Values for the Sun, Moon, Earth, Venus and Mars are unaffected.
Slight correction: 22.546 degrees is more accurate. I should also point out that the Earth is oblate, rather than perfectly round (a point avoided above!|).
Lastly, for a scholarly (and very esoteric) "take" on the subject see Richard Oosterhoff: https://www.research.ed.ac.uk/en/publications/learned-failure-and-the-untutored-mind-emblem-21-of-atalanta-fugi
geoffss@aol.com
perpetual choirs has moved to https://perpetualchoirs.webador.co.uk/home
tarot II has moved to https://free-5167342.webadorsite.com/
By-the-by, a curiosity linked to Frank C Higgins, the Great Pyramid and the Masonic Apron.
Using our 11 x 11 grid above we draw this:
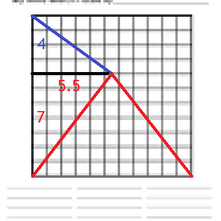

Now Higgins claimed that the masonic apron contains the maths of the Great Pyramid slope*. This is circa 51 51 degrees, tan 1.273. 7/5.5, above, gives a close value of tan 1.2727r, noting reciprocal of true pi/4 = 1.2732. He further claimed the apron generated a 3-4-5 triangle from this. But 5.5/4 = 1.375. Tan 54 is 1.376** whilst tan 4/3 = 1.333 (this being the equivalent 3-4-5 angle). So the discovered triangle above is much more a 36-54-90 than a 3-4-5. This 36-54-90 is the maths of pentagons and phi. And it is nothing like the actual apron shown on the right, is it? And aprons vary, anyway (with virtually none I can find being square - which is what you'd need to deliver the maths). However, in 1919, Higgins was using 51.5 for the Great Pyramid. Now this generates tan 1.346-ish - much closer to the 3-4-5 angle 1.333 with a square to work from. See https://archive.ph/x0UHx
**Using true pi we get 1.37589-ish - pretty damn close!.
And one last point (punning a tad): If the Maier compass point rests on Earth circle centre then a vertical through it would not seem to go through the top of the pyramid ... Oh, well ...
e&oe

Create Your Own Website With Webador